ChtMultiRegionFoam
ChtMultiRegionFoam
Solver for steady or transient fluid flow and solid heat conduction, with conjugate heat transfer between regions, buoyancy effects, turbulence, reactions and radiation modelling.
Contents
1 Equations
For each region defined as fluid, the according equation for the fluid is solved and the same is done for each solid region. The regions are coupled by a thermal boundary condition.
1.1 Equations Fluid
For each fluid region the compressible Navier Stokes equation are solved.
1.1.1 Mass conservation
The variable-density continuity equation is
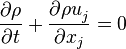 | (1) |
The source code can be found in src/finiteVolume/cfdTools/compressible/rhoEqn.H:
{ fvScalarMatrix rhoEqn ( fvm::ddt(rho) + fvc::div(phi) == fvOptions(rho) ); fvOptions.constrain(rhoEqn); rhoEqn.solve(); fvOptions.correct(rho); }
1.1.2 Momentum conservation
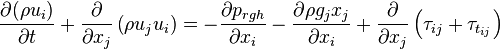 | (2) |
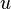 represent the velocity,
represent the velocity, 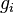 the gravitational acceleration,
the gravitational acceleration, 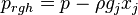 the pressure minus the hydrostatic pressure and
the pressure minus the hydrostatic pressure and
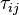 and
and 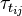 are the viscose and turbulent stresses.
are the viscose and turbulent stresses.
The source code can be found in Ueqn.H:
// Solve the Momentum equation
MRF.correctBoundaryVelocity(U);
tmp<fvVectorMatrix> tUEqn
(
fvm::ddt(rho, U) + fvm::div(phi, U)
+ MRF.DDt(rho, U)
+ turbulence.divDevRhoReff(U)
==
fvOptions(rho, U)
);
fvVectorMatrix& UEqn = tUEqn.ref();
UEqn.relax();
fvOptions.constrain(UEqn);
if (pimple.momentumPredictor())
{
solve
(
UEqn
==
fvc::reconstruct
(
(
- ghf*fvc::snGrad(rho)
- fvc::snGrad(p_rgh)
)*mesh.magSf()
)
);
fvOptions.correct(U);
K = 0.5*magSqr(U);
}
fvOptions.correct(U);
1.1.3 Energy conservation
The energy equation can be found in: https://cfd.direct/openfoam/energy-equation/
The total energy of a fluid element can be seen as the sum of kinetic energy 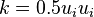 and internal energy
and internal energy  .
The rate of change of the kinetic energy within a fluid element is the work done on this fluid element by the viscous forces, the pressure and eternal volume forces like the gravity:
.
The rate of change of the kinetic energy within a fluid element is the work done on this fluid element by the viscous forces, the pressure and eternal volume forces like the gravity:
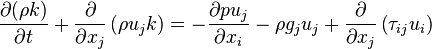 | (3) |
The rate of change of the internal energy  of a fluid element is the heat transferred to this fluid element by diffusion plus the
heat source term
of a fluid element is the heat transferred to this fluid element by diffusion plus the
heat source term  plus the heat source by radiation
plus the heat source by radiation 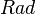 :
:
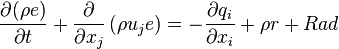 | (4) |
The change rate of the total energy is the sum of the above two equations:
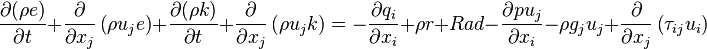 | (5) |